Maximum Subarray Problem

Problem Description

Input: An array A[1,…,n] consists of integer elements Output: An array A[i,…,j], it is a subarray of A[1,…,n] and the sum of its elemments are the biggest among all subarrays

Solution 1. Brute-force

The Brute-force method simply traverse all subarrays of A[1,..,n] to find the maximum subarray. It takes $\Theta (n^2)$ time.

Solution 2. Divide and Conquer

This method can be viewed in Introduction to Algorithms.

The algorithm above can be further improved by not searching each entire half array to find maximum cross-sum. We can stop when we go beyond the end of max-subarray in each half. This is because when our array has included the max-subarray in this half, our array will never be greater than the max-subarray. So there is no need to continue iterating.

Solution 3. Scanning

This method is based on the fact that if max subarray for $A[1,…,j]$ is already known as $A_{max}$, then new $A_{max}$ in $A[1,…,j+1]$ must be previous $A_{max}$ or subset in the form of $A[k,…,j+1]$. And we can use information from last round of iteration to find the new $A_{max}$ in constant time.
Then the biggest question is: what information should we record in a round of iteration? The answer is : we should keep the sum of $A[i,…,j]$(which is called $temp$), where $i$ is the head of current max subset. Then we can compare $sum$ with $temp+A[j+1]$ to determine if we have found a new max subset and should update. If not, we should examine if $temp$ is below zero. Why? It is because if $temp$ is negative, it will not contribute to $A[j+1]$ or say $A[j+1]$ will definitely be less than $A[j+1]+temp$. Also, we no longer need to keep previous sum of $A[i,…,j]$ or say $temp$ anymore. Instead, we use $A[j+1]$ to update $temp$. This means re-accumulate $temp$ and prepare for the calculation of sum of $temp+A[j+1]$ and comparation, which can be finished within $O(n)$ time.

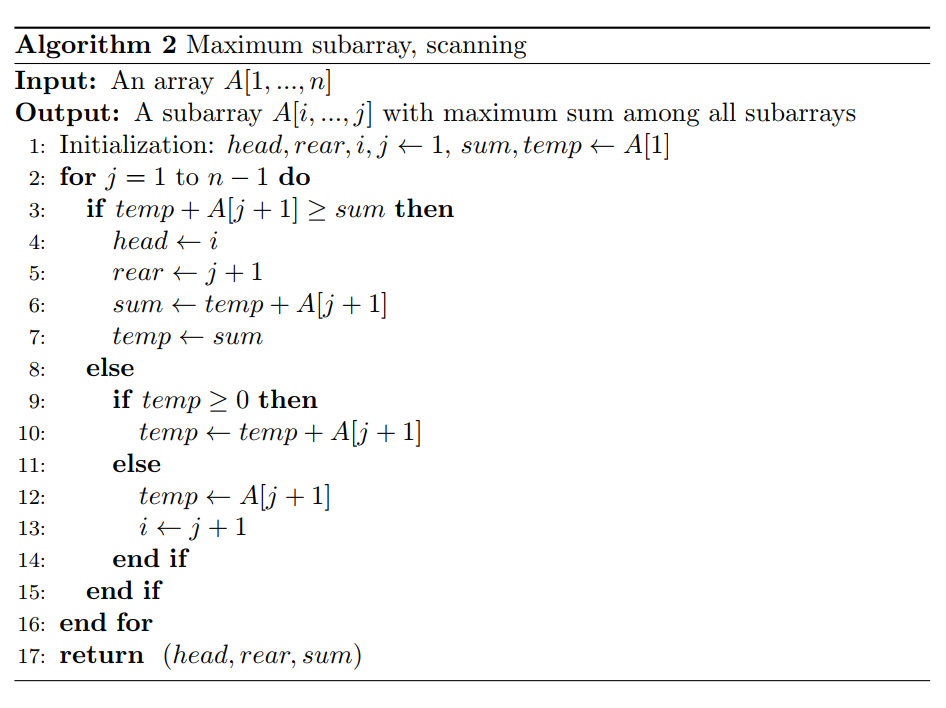
The code for scanning method can be view here.